Infinite dimensions have long fascinated mathematicians, challenging their understanding and igniting their imaginations. Conveying the depths of this abstract concept in a tangible form is no easy task, yet the idea of a math-themed lamp seeks to do just that. This article explores the quest to find the perfect base shape that can represent infinite dimensions in a visually captivating manner, captivating both mathematicians and enthusiasts alike.
The notion of infinity permeates various mathematical disciplines, from calculus to geometry, and holds a profound significance in our understanding of the universe. However, visually representing such an intangible and boundless concept poses a unique challenge. By exploring the symbolic power of shapes, the characteristics of geometric design, and the possibilities of abstract representation, we can begin to unravel the secrets of visually capturing infinite dimensions in the form of a math-themed lamp.
Defining Infinity in Mathematics
Infinity, a concept rooted in mathematics, represents the idea of unboundedness and endlessness. It exists beyond the constraints of finite numbers and has fascinated mathematicians for centuries. Whether contemplating the infinite series in calculus or pondering the infinite sets in set theory, mathematicians have grappled with its complexities.
Visually representing infinity poses a unique challenge. How can something that knows no bounds be captured within the confines of a physical object? The aim of a math-themed lamp is to bridge this gap and create a visual representation that alludes to the vastness of infinite dimensions.
The Challenges of Representation
One of the primary challenges in representing infinity visually is the difficulty of comprehending such an expansive concept. Infinity surpasses human perception and stretches the limits of our understanding. However, by leveraging the power of symbolism and utilizing shapes that evoke association with infinity, we can create a visual language that alludes to its abstract nature.
Let’s delve into the world of shapes and explore how they can become the building blocks for representing infinite dimensions in the design of a math-themed lamp.
The Symbolism of Shapes
Shapes hold immense significance in various cultures and industries, symbolizing different concepts and ideas. From the sacred geometry of ancient civilizations to contemporary design, shapes communicate meaning and evoke emotions. By carefully selecting a base shape for the math-themed lamp, we can tap into this rich symbolic language to convey the essence of infinite dimensions.
Shapes Associated with Infinity
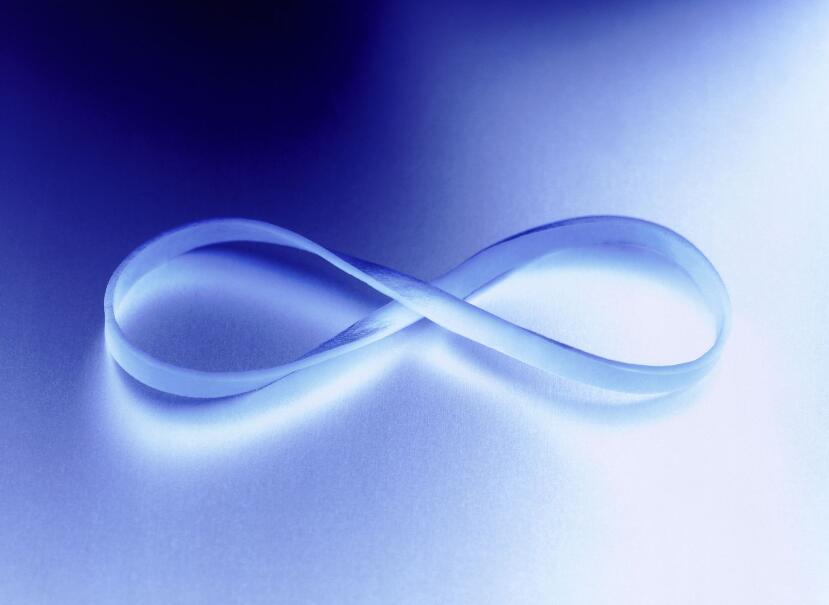
Throughout history, mathematicians and artists have explored shapes that embody the concept of infinity. One such shape is the Möbius strip, a surface with only one side and one boundary. Its endless loop captures the eternal nature of infinite dimensions.
Fractals are another fascinating example of shapes associated with infinity. These intricate patterns exhibit a self-repeating property at different scales. A fractal-based design for the lamp base would convey the idea of infinity through the infinite complexity found within a finite form.
While these shapes have traditionally been linked to the concept of infinity, it’s important to examine other geometric considerations to discover an even more compelling base shape.
Geometric Considerations
Geometry provides a wealth of possibilities when it comes to representing infinite dimensions. The inherent properties and visual qualities of geometric shapes can evoke a sense of boundlessness and unending expansion.
Exploring Circular and Spiral Shapes
Circles hold a special place in mathematics and can be seen as a symbol of perfection and unity. Their infinite number of points around a central locus suggests a continuum without boundaries. A base shape inspired by circles could capture the essence of infinite dimensions by visually referencing their unbounded nature.
Spirals, on the other hand, offer a captivating representation of growth and expansion. From the infinitely expanding spiral of the Fibonacci sequence to the logarithmic spirals found in nature, these shapes evoke a sense of limitless growth that resonates with the concept of infinite dimensions.
By carefully considering the symbolism and properties of these shapes, we can begin to narrow down our search for the ideal base shape for the math-themed lamp.
When exploring circular shapes, one possibility is to design the lamp base in the form of a perfect circle. A circle represents unity, completeness, and infinite symmetry. Its continuous and unbroken boundary suggests a seamless connection with no beginning or end. By incorporating a circular base, the math-themed lamp would visually evoke the boundless nature of infinite dimensions.
Spirals, with their captivating and dynamic form, provide another avenue for representing infinite dimensions. These shapes exhibit a progressive expansion while maintaining a consistent pattern. The Fibonacci spiral, derived from the famous Fibonacci sequence, demonstrates a logarithmic growth found in nature. By incorporating a spiral shape in the lamp base design, the visual representation would evoke the sense of continuous growth and unending progression akin to infinite dimensions.
Both circular and spiral shapes offer unique opportunities for visually capturing the concept of infinite dimensions in a math-themed lamp. By carefully studying their symbolism and properties, designers can choose a base shape that best resonates with the essence of infinity.
Abstract Representations
While geometric shapes provide tangible representations of infinity, exploring abstract shapes allows for even more innovative and thought-provoking designs for the math-themed lamp. These unconventional shapes push the boundaries of imagination and challenge traditional notions of form and structure.
Hypercubes: Embracing Higher Dimensions
A hypercube, also known as a tesseract, is a four-dimensional analog of a cube. Although it may be challenging to visualize, it offers a fascinating representation of additional dimensions beyond our three-dimensional reality. By incorporating a hypercube-inspired base shape, the math-themed lamp would promote the exploration of higher dimensions and spark conversations about the nature of infinity.
Klein Bottles: Blurring Boundaries
The Klein bottle is a non-orientable surface with no distinct “inside” or “outside.” It is a truly unique shape that challenges traditional notions of space and dimensionality. By incorporating the intricate and complex form of a Klein bottle into the design of the lamp base, it would serve as a visual metaphor for the boundless and unbounded nature of infinite dimensions.
Embracing abstract shapes requires a delicate balance. Designers must consider the lamp’s functionality, structural integrity, and the ability to convey the concept of infinite dimensions in a visually pleasing manner. However, by pushing the boundaries and exploring unconventional shapes, we can create lamps that truly embody the enigmatic nature of infinity.
Practical Design Considerations
While the quest to find the perfect base shape to represent infinite dimensions is fascinating, practical considerations cannot be overlooked. A math-themed lamp not only needs to visually allude to infinity but also needs to function as a source of illumination and complement its surroundings in a thoughtful and appealing manner.
Finding a Balance Between Complexity and Simplicity
When designing the math-themed lamp, finding the right balance between complexity and simplicity is crucial. The base shape should be visually captivating and convey the concept of infinite dimensions, but it should not overwhelm the overall aesthetic or distract from its purpose as a practical lighting fixture.
Simplicity in design can often be more effective in conveying the essence of infinity. A minimalist approach, with clean lines and a streamlined form, can evoke a sense of elegance and timelessness. By using a simple yet carefully crafted base shape, the math-themed lamp can capture the essence of infinite dimensions without becoming visually overpowering.
Materials and Finishes
The choice of materials and finishes is another important consideration in the design of the math-themed lamp. The materials should be durable and of high quality to ensure the longevity of the lamp. Additionally, the finish should enhance the overall aesthetic and contribute to the representation of infinite dimensions.
Reflective surfaces can create an illusion of depth and expansiveness, symbolizing the infinite expanse of dimensions. A polished metal finish or a mirrored surface can give the base shape a sense of endlessness and add a touch of modern elegance to the lamp.
Alternatively, a translucent or frosted material can be used to create a diffused light effect, giving the lamp an ethereal quality. This effect can represent the intangible and elusive nature of infinity, casting a soft and captivating glow that enhances the overall ambiance of the space.
Conclusion
The quest to visually represent infinite dimensions through a math-themed lamp is a combination of mathematical exploration, design creativity, and technological innovation. By carefully selecting a base shape that embodies the symbolism and properties associated with infinity, designers can create lamps that intrigue and inspire.
From exploring geometric shapes like circles and spirals to embracing abstract representations such as hypercubes and Klein bottles, each shape offers a unique perspective on the concept of infinity. The challenge lies not only in finding a visually captivating base shape but also in considering practical design elements like simplicity, materials, and finishes.
Ultimately, a math-themed lamp has the power to evoke a sense of wonder and curiosity, reminding us of the limitless possibilities and unexplored dimensions that exist beyond our comprehension. It serves as a tangible reminder of the beauty and intricacy of mathematics and its ability to transcend boundaries and connect us with the infinite.